|
Ya i know how lame am i right? asking for math help here. Buty i knww most of you are the some of the smartest fucks so if you could help me that would be great.
I really just need t know the formula for the Volume and Surface are for a pyramid and a cone. If i can get that down i can do it. If i know all the steps.
Volume isnt so bad its the Surface area i cant remember. But id still like help with both.
Here a pyramid i need help with:
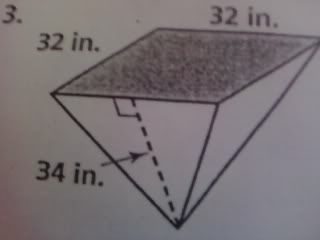
and Heres a Cone:
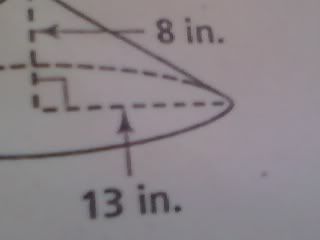
Help me out please. Ive been needing these who a long time now. I dont got many problems 12 but somes already done, and thats the ones we went over and i stapled my notes to another paper when i turned it in.
Make awkward sexual advances, not war. Down Rodeo said: Dammit, this was the one place that didn't have this, but noooooo, molkman pisses all over that 
|
|
|
|
|
≡
|
2010 Jan 31 at 22:16 UTC
— Ed. 2010 Jan 31 at 22:17 UTC

|
|
|
|
I believe, volume is base X width X height. Right?
|
|
|
|
|
≡
|
2010 Jan 31 at 22:21 UTC

|
|
|
Down Rodeo
Cap'n Moth of the Firehouse


2007 Oct 19 • 5486
57,583 ₧
|
That'd be for a cube. Surface area is easy, just add the areas of the faces. The volume of a cone... I'd need to think. Technically you can do it by volume integration but I think your best bet is wikipedia.
|
|
|
|
|
≡
|
2010 Jan 31 at 22:27 UTC

|
|
|
|
All i know is the cone involved alot of pi r squared and there's so many different formulas it gives me a brain fart.
Volume for a Triangle is bh/2 X H and surface area for it is 2(Area of the triangle)(bh/2) + the sides squared. Sadly these arent Triangles.
Make awkward sexual advances, not war. Down Rodeo said: Dammit, this was the one place that didn't have this, but noooooo, molkman pisses all over that 
|
|
|
|
|
≡
|
2010 Jan 31 at 22:34 UTC

|
|
|
|
SolidKAYOS said: pi r squared
PI R ROUND!!!
I drink to forget but I always remember.
|
|
|
|
|
≡
|
2010 Feb 1 at 03:28 UTC

|
|
|
Rockbomb
Dog fucker (but in a good way now)

2009 Nov 14 • 2045
|
the_cloud_system said: SolidKAYOS said: pi r squared
PI R ROUND!!! Lmfao...
For the pyramid, get the area of the square side (length times width), then get the area of one of the triangular sides (width times height divided by 2) and multiply that by four, then add whatever you got for the area of the square to that.
For the cone, read this link http://math.about.com/od/formulas/ss/surfaceareavol_2.htm
|
|
|
|
|
≡
|
2010 Feb 1 at 03:55 UTC

|
|
|
|
Volume of Pyramid = ((32in * 32in) * Height) / 3
You can get the height using Pythagoras' theorem:
Height^2 = (34in)^2 - (16in)^2
Volume of Cone = ((pi * (13in)^2) * 8in) / 3
Surface of Pyramid = Base(see above) + 4 * (32in * 34in) / 2 = Base + 2 * 32in * 34in
Surface of Cone = Base(see above) + (pi * 13in * s)
You can get s again using Pythagoras' theorem:
s^2 = (8in)^2 + (13in)^2
Hopefully all of the above is correct.
...and that's the bottom line because Mate de Vita said so.
|
|
|
|
|
≡
|
2010 Feb 1 at 12:12 UTC

|
|
|
|
Mate de Vita said: Volume of Pyramid = ((32in * 32in) * Height) / 3
You can get the height using Pythagoras' theorem:
Height^2 = (34in)^2 - (16in)^2
Volume of Cone = ((pi * (13in)^2) * 8in) / 3
Surface of Pyramid = Base(see above) + 4 * (32in * 34in) / 2 = Base + 2 * 32in * 34in
Surface of Cone = Base(see above) + (pi * 13in * s)
You can get s again using Pythagoras' theorem:
s^2 = (8in)^2 + (13in)^2
Thank you. That seems about right. Now im ganna go do it. Thanks for all who helped. Ill ask if theres any other troubles i run into.
Hopefully all of the above is correct.
Make awkward sexual advances, not war. Down Rodeo said: Dammit, this was the one place that didn't have this, but noooooo, molkman pisses all over that 
|
|
|
|
|
≡
|
2010 Feb 1 at 23:44 UTC

|
|
|
aaronjer
*****'n Admin


2005 Mar 21 • 5174
1,227 ₧
|
Why did you put your response in the middle of a quote of Mate's post? 
|
|
|
|
|
≡
|
2010 Feb 2 at 06:06 UTC

|
|
|
|
Oh O.o Typo i guess haha
Make awkward sexual advances, not war. Down Rodeo said: Dammit, this was the one place that didn't have this, but noooooo, molkman pisses all over that 
|
|
|
|
|
≡
|
2010 Feb 2 at 09:20 UTC

|
|
|
aaronjer
*****'n Admin


2005 Mar 21 • 5174
1,227 ₧
|
But... how would... how did...? Damn, you really can't see for shit, can you.
|
|
|
|
|
≡
|
2010 Feb 2 at 15:34 UTC

|
|
|
|
aaronjer said: But... how would... how did...? Damn, you really can't see for shit, can you.

...and that's the bottom line because Mate de Vita said so.
|
|
|
|
|
≡
|
2010 Feb 2 at 16:06 UTC

|
|
|
|
aaronjer said: But... how would... how did...? Damn, you really can't see for shit, can you.
Nope lmao
Make awkward sexual advances, not war. Down Rodeo said: Dammit, this was the one place that didn't have this, but noooooo, molkman pisses all over that 
|
|
|
|
|
≡
|
2010 Feb 2 at 21:27 UTC

|
|
|
|
Mate de Vita said: Volume of Pyramid = ((32in * 32in) * Height) / 3
You can get the height using Pythagoras' theorem:
Height^2 = (34in)^2 - (16in)^2
Volume of Cone = ((pi * (13in)^2) * 8in) / 3
Surface of Pyramid = Base(see above) + 4 * (32in * 34in) / 2 = Base + 2 * 32in * 34in
Surface of Cone = Base(see above) + (pi * 13in * s)
You can get s again using Pythagoras' theorem:
s^2 = (8in)^2 + (13in)^2
Hopefully all of the above is correct.
You surface area for them kinda confused the crap out of me. But i figured it out.
SA of Cone= pi * r * s + pi * r^2
SA of Pyramid= 2bs+b^2
Make awkward sexual advances, not war. Down Rodeo said: Dammit, this was the one place that didn't have this, but noooooo, molkman pisses all over that 
|
|
|
|
|
≡
|
2010 Feb 7 at 19:24 UTC
— Ed. 2010 Feb 7 at 19:25 UTC

|
|
|
|
Page [1]
|